Question

If f(x) is an exponential function where f(3.5)=16 and f(4)=99, then find the value of f(6), to the nearest hundredth.

Answer 1

To find the value of f(6), you can use the exponential function given with two points f(3.5)=16 and f(4)=99. Solve the equations to find the values of a and b, then substitute x=6 to find f(6) to the nearest hundredth.

Step-by-step explanation:

To find the value of f(6), we can use the exponential function given with two points f(3.5) = 16 and f(4) = 99.

Let's assume the exponential function is of the form f(x) = a * b^x. We can use the two points to form two equations:

`16 = a * b^(3.5)`

`99 = a * b^4`

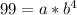
We can solve these equations to find the values of a and b. Then we can substitute x = 6 into the exponential function to calculate f(6) to the nearest hundredth.

The value of f(6) is approximately 160.72.