Answer:
length = 4 yards , width = 3.5 yards
Explanation:
assuming you require the dimensions of length and width of the rectangle.
let w be the width , then length = 2w - 3 ( 3 less than twice the width )
the area (A) of a rectangle is calculated as
A = length × width
given A = 14 , with width w and length 2w - 3
substitute these values into the formula for A
w(2w - 3) = 14 ← distribute parenthesis on left side
2w² - 3w = 14 ( subtract 14 from both sides )
2w² - 3w - 14 = 0 ← quadratic equation in standard form
consider the factors of the product of the coefficient of the w² term and the constant term which sum to give the coefficient of the w- term.
product = 2 × - 14 = - 18 and sum = - 3
the factors are + 4 and - 7
use these factors to split the w- term
2w² + 4w - 7w - 14 = 0 ( factor the first/second and third/fourth terms )
2w(w + 2) - 7(w + 2) = 0 ← factor out (w + 2) from each term
(w + 2)(2w - 7) = 0 ← in factored form
equate each factor to zero and solve for w
w + 2 = 0 ( subtract 2 from each side )
w = - 2
2w - 7 = 0 ( add 7 to both sides )
2w = 7 ( divide both sides by 2 )
w =
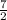 = 3.5
= 3.5
and length = 2w - 3 = 2(3.5) - 3 = 7 - 3 = 4
Then length = 4 yards and width = 3.5 yards