Question

The following two-way table describes students' after school activities. Find the probability that a randomly selected student is in sports, given that it's a senior. A. 0.25 B. 0.40 C. 0.60 D. 0.75

Answer 1

The probability that a randomly selected student is in sports, given that they are a senior, is approximately 0.71, rounded to the nearest hundredth.

Add up the values in the "Sports," "Music/Drama," and "Work" columns for the senior row:
`$25+5+5=35$`
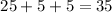
From the table, we see that there are 25 senior students in sports.

`P(\text { Sports } \mid \text { Senior })=\frac{\text { Number of Senior Students in Sports }}{\text { Total Number of Senior Students }}`

`P(\text { Sports } \mid \text { Senior })=(25)/(35)`

`P(\text { Sports } \mid \text { Senior }) \approx (25)/(35) \approx 0.7143`

`P(\text { Sports } \mid \text { Senior }) \approx 0.71`

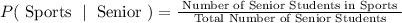
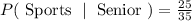
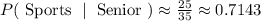
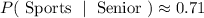
Complete Question:

The following two-way table describes students after. school activities. Find the probability that a randomly selected student is in sports, given that it's a senior.

Grade Sports Music/Drama Work

Sophomore 20 7 3

Junior 20 13 2

Senior 25 5 5

P(Sports|Senior) = [?]

Round to the nearest hundredth.