Given,
The initial velocity of the ball, u=2.3 m/s
The time interval in which the ball hits the ground, t=4 s
As the ball was thrown horizontally, the angle of projection is θ=0°
The distance traveled by the ball in the horizontal direction is given by,
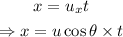
Where uₓ is the x-component of the initial velocity.
On substituting the known values,
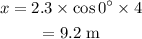
Therefore the distance traveled by the ball in the x-direction is 9.2 m. That is the ball landed 9.2 meters away from the building.
Applying the equation of the motion in the y-direction,

Where y is the final height of the ball which is zero meters, y₀ is the initial height of the ball, i.e., the height of the building, uy is the y-component of the initial velocity.
Let us consider that the upward direction is positive while the downward direction is negative. This makes the acceleration due to gravity, g, a negative value, and the height of the building a positive value.
On substituting the known values,
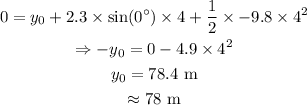
Therefore the height of the building is 78 m