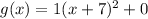Answer:
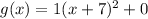
Explanation:
Given
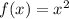
Required
Determine g(x) where g(x) is a translation of f(x), 7 units to the right
When a point is translated to the right, the resulting point is:
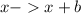
Where b is the number of units to the right.
In this case:
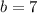
So, we have:
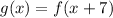
Solving f(x+7)
Substitute x + 7 for x in
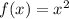
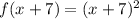
So, we have:
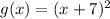
To write in the form of
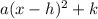 , we have:
, we have: