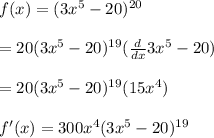Question

F(x)=(3x^5-20)^20 Find the derivative

Answer 1

`f'(x)=300x^4(3x^5-20)^(19)`

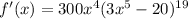
Explanation:

There are two derivative rules that are present in this problem: the chain rule and the power rule.

Since the given function is a composition of two functions, we must use the chain rule to find the derivatives of both the inside and the outside of the functions.

When solving for the derivative of the inside function, the power rule is necessary.

`f(x)=(3x^5-20)^(20)\\\\=20(3x^5-20)^(19) ((d)/(dx)3x^5-20)\\\\=20(3x^5-20)^(19)(15x^4)\\\\f'(x)=300x^4(3x^5-20)^(19)`