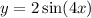The equation of a sine function with an amplitude of 2 and a period of 4π is represented as
 . (option D)
. (option D)
Why is this correct?
The equation of a sine function with an amplitude
 and a period (\
and a period (\
 is given by:
is given by:
![\[ y = A \sin\left((2\pi)/(P)x\right) \]](https://img.qammunity.org/2024/formulas/physics/high-school/alpc5hmru19prcis5xdw0cehyex6zmbg2g.png)
Given:
Amplitude
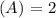
Period
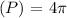
Substituting these values into the equation:
![\[ y = 2 \sin\left((2\pi)/(4\pi)x\right) \]](https://img.qammunity.org/2024/formulas/physics/high-school/l6mn8s9inbj75iwr7h6n7pjlbs75n6e9lu.png)
![\[ y = 2 \sin\left((1)/(2)x\right) \]](https://img.qammunity.org/2024/formulas/physics/high-school/haa4xdelei7n6se4gozeaqm4gajxrij6vd.png)
Comparing this equation with the provided options:
A)
 - This has an amplitude of 2 but a different period not
- This has an amplitude of 2 but a different period not
 ).
).
B)
 - This has a different amplitude and a period of
- This has a different amplitude and a period of
 , not
, not
 .
.
C)
 - This has the correct amplitude of 2 but a period of
- This has the correct amplitude of 2 but a period of
 , not
, not
 .
.
D)
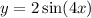 - This has both the correct amplitude of 2 and the correct period of
- This has both the correct amplitude of 2 and the correct period of
 .
.
Therefore, the correct answer is: D)