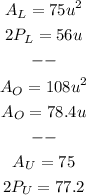Question

I need help with finding the area and perimeter of the letter o

Answer 1

Check below, please.

1) In this question, we're going to remember two concepts: The perimeter is the sum of the lengths of each segment of each letter.

2) So let's start counting each tiny square so that we can get to know the length.

The letter "L" is actually, with this typography, two rectangles:

So, the perimeter (2P) is equal to:

2P =15 +15 +7+3+3+10+3

2P= 56 units

As for the area:

Using the Rectangle formula, then we can write down the area as:

Area:

`\begin{gathered} A=l\cdot w \\ A_1=3\cdot15=45u^2 \\ A_2=10\cdot3=30u^2 \\ A_L=30+45=75u^2 \end{gathered}`

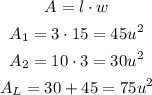
3) In this letter "O" we can divide it into two trapezoids, and two parallel rectangles:

Note that we need to find the length of those corners shaped like triangles, we can use the Pythagorean Theorem, considering the "rise over run" and write:

`\begin{gathered} a^2=3^2+2^2 \\ a^2=9+4 \\ a^2=13 \\ \sqrt[]{a^2}=\sqrt[]{13} \\ a=3.6 \end{gathered}`

So the Perimeter can be written:

`\begin{gathered} 2P=3.6+3.6+3.6+3.6+5+5+12+12+12+12+3+3 \\ 2P_O=78.4 \end{gathered}`

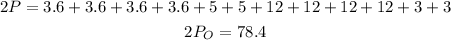
And for the area, we can find the area of those two trapezoids and two rectangles writing this:

`\begin{gathered} A_O=2(((B+b)h)/(2))+2(w* l) \\ A_O=2(((9+3)3)/(2))+2(12*3)_{} \\ A_O=108u^2 \end{gathered}`

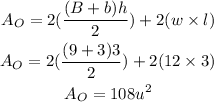
4) And now, finally the letter "u":

For the corners let's assume they are triangles, and then we can write the following since those corners are like hypotenuses:

`\begin{gathered} a^2=5^2+2^2 \\ a^2=25+4 \\ a=\sqrt[]{29}\approx5.4 \end{gathered}`

And for the inclined lower part of the letter "u", we can write:

`\begin{gathered} a^2=1^2+2^2 \\ a=\sqrt[]{5}\approx2.2 \end{gathered}`

Therefore, we can write the Perimeter as:

`\begin{gathered} 2P=2(5.4)+2(2.2)+4+3(2)+4(13) \\ 2P_U=77.2 \end{gathered}`

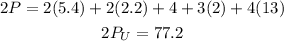
And for the area, we can see from bottom to top: One trapezoid, a par of parallelograms, and two rectangles. Hence, we can write:

`\begin{gathered} A_U=((B+b)h)/(2)+2(l\cdot w)+2(l\cdot w) \\ A_U=((6+4)3)/(2)+2(2\cdot2)+2(2\cdot13) \\ A_U=75u^2 \end{gathered}`

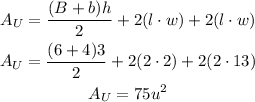
5) So, each letter by area and perimeter:

`\begin{gathered} A_L=75u^2 \\ 2P_L=56u \\ -- \\ A_O=108u^2 \\ A_O=78.4u \\ -- \\ A_U=75 \\ 2P_U=77.2 \end{gathered}`