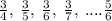Question

List three rational numbers between 3/4 and 5/7

​

Answer 1

3/5, 3/6, 5/6.....

Explanation:

The first step to execute as finding the three rational numbers, let's first understand what a rational number is;

• What is a rational number?

A rational number is a number that can be expressed as a fraction whose numerator (top number) and denominator (bottom number) are both integers, and it's denominator is not equal to zero

`{ \rm{ i.e : \: \: \: \: (a)/(b) }} \\ \\ { \rm{ - \: where \: a \: and \: b \: are \: integers}} \\ { \rm{ - \: b≠0 \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: \: }}`

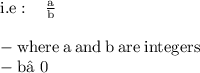
That means both 3/4 and 5/7 are all rational numbers

• Choosing rational numbers

So, any fraction lying between 3/4 and 5/7 is a rational number.

`{ \rm{ (3)/(4), \: (3)/(5), \: (3)/(6), \: (3)/(7), \: .... (5)/(6) }} \\`