Answer:
>>>
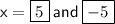
Explanation:
Given that ,
We have to find ,
Solution :
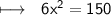
Step 1 : Dividing both sides with 6 :
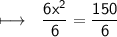
We get ,

Step 2 : Taking square root on both sides :

We have ,
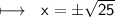

- Therefore , value of x are " +5 and -5 "
Verification :-
#When x = 5 :
#When x = -5 :
Therefore , our answer is correct
- Hope, it'll help you!! :)