Question

The first term of an arithmetic sequence is 5, and the commen difference is 3. What is the 20th term of sequence

Answer 1

• the 20th term of the sequence is 62.

Explanation:

Given:

• The first term of an arithmetic sequence is 5
• The commen difference is 3

The formula of nth term is given by:

• 
  `{ \boxed{\rm{a_n = a+(n-1)d}}}`

here,

• “a” is first term
• n is number of terms. Here in the question we are asked to find the 20th term of the sequence, so the value of n will be 20
• “d” is common difference.

On putting the required values,

`\rm a_(20) = 5+(20-1)3 \\ \\ \rm \: a_(20) = 5+(19)3 \\ \\ \rm \: a_(20) = 5 + 57 \\ \\ \: { \boxed{\rm{ \red{a_(20) =62}}}}`

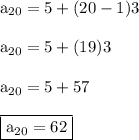
Hence, the 20th term of the sequence is 62.

`\dotfill`