Question

Write an expression in factored form for the polynomial of least possible degree graphed below.

Answer 1

Answer: (1/32)(x-4)(x+4)(x-2)(x+2)

This is the same as writing
`(1)/(32)(\text{x}-4)(\text{x}+4)(\text{x}-2)(\text{x}+2)`

The order of the factors does not matter.

===================================================

Explanation

The roots or x intercepts shown are: -4, -2, 2, and 4.

Let's rearrange them a bit to write: -4, 4 and -2, 2

The -4, 4 portion gives (x-4)(x+4).

The -2, 2 portion gives (x-2)(x+2).

Overall the factored form would be (x-4)(x+4)(x-2)(x+2).

However, expanding that out gives us

(x-4)(x+4)(x-2)(x+2) = (x^2-16)(x^2-4) = x^4 - 20x^2 + 64

The last term, aka the constant term, 64 should be 2 because the y intercept of the graph is 2.

To go from 64 to 2, we multiply by 1/32.

That must mean we need 1/32 as the leading coefficient out front.

That gives the final answer (1/32)(x-4)(x+4)(x-2)(x+2)

Answer 2

`y(x)=(1)/(32)(x+2)(x-2)(x+4)(x-4)`

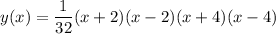
Explanation:

The root of a polynomial is a point on the graph where the curve intersects or crosses the x-axis, meaning that the function equals zero at that point.

From observation of the given graph, the roots of the polynomial are:

• x = -4
• x = -2
• x = 2
• x = 4

The curve exhibits symmetry about the y-axis.

According to the Factor Theorem, if a polynomial f(x) has a root at x = a, then (x - a) is a factor of f(x).

The multiplicity of a root refers to the number of times the associated factor appears in the factored form of the equation of a polynomial.

When a root has a multiplicity of one, the graph of the polynomial function crosses the x-axis at that particular value of x. The concavity of the graph remains the same as it approaches and passes through the x-axis. Therefore, all roots of the graphed function have multiplicity one.

So, a possible factored form of the graphed function is:

`y(x)=a(x+4)(x+2)(x-2)(x-4)`

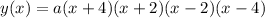
where 'a' is the leading coefficient.

The y-intercept is the point on the graph where the curve intersects the y-axis. Therefore, the y-intercept is at (0, 2).

To find the leading coefficient (a), we can substitute (0, 2) into the function and solve for a:

`\begin{aligned}y(0)=a(0+4)(0+2)(0-2)(0-4)&=2\\a(4)(2)(-2)(-4)&=2\\64a&=2\\a&=(1)/(32)\end{aligned}`

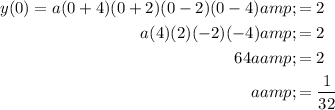
So, the equation of the graphed function in factored form is:

`y(x)=(1)/(32)(x+4)(x+2)(x-2)(x-4)`

`y(x)=(1)/(32)(x+2)(x-2)(x+4)(x-4)`

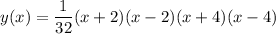
This equation satisfies the observed characteristics of the graph.