Question

The mass of the particles that a river can transport is proportional to the fifth power of the speed of the river. A certain river normally flows at

a speed of 4 miles per hour. What must its speed be in order to transport particles that are 12 times as massive as usual? Round your
answer to the nearest hundredth.

Answer 1

6.58 miles per hour

Explanation:

Let the mass and speed be represented by m and s respectively. So that,

m
`\alpha`
`s^(5)`

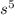
m = k
`s^(5)`

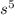
where k is the constant of proportionality.

For a certain river, speed = 4 miles per hour.

m = k
`(4)^(5)`

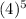
m = 1024k ............ 1

In order to transport particles that are 12 times as massive as usual;

12m = k
`s^(5)`

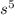
m =
`(ks^(5) )/(12)` ............. 2

Thus,

`(ks^(5) )/(12)` = 1024 k

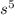`s^(5)` = 12 x 1024

= 12288

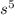`s^(5)` 12288

s =
`\sqrt[5]{12288}`

= 6.5750

s = 6.58 miles per hour

Therefore, its speed must be approximately 6.58 miles per hour.