Question

What is dy/dx of 2^xy = 1?

Answer 1

`\frac{\text{d}y}{\text{d}x}=-(y)/(x)`

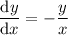
Explanation:

Given equation:

`2^(xy)=1`

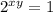
To differentiate the given equation, begin by taking the natural logarithm (ln) of both sides:

`\ln \left(2^(xy)\right)=\ln(1)`

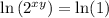
Apply the power law of logarithms to the left side, and the logarithmic property ln(1) = 0 to the right side:

`xy\ln(2)=0`

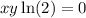
To differentiate an equation that contains a mixture of x and y terms, we can use implicit differentiation.

Begin by placing d/dx in front of each term of the equation:

`\frac{\text{d}}{\text{d}x}\left(xy\ln(2)\right)=\frac{\text{d}}{\text{d}x}(0)`

Differentiate the constant term:

`\frac{\text{d}}{\text{d}x}\left(xy\ln(2)\right)=0`

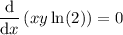
Use the product rule to differentiate the term in x and y.

`\boxed{\begin{array}{l}\underline{\sf Product\;Rule\;for\;Differentiation}\\\\\textsf{If}\;y=uv\;\textsf{then:}\\\\\frac{\text{d}y}{\text{d}x}=u\frac{\text{d}v}{\text{d}x}+v\frac{\text{d}u}{\text{d}x}\\\\\end{array}}`

`\textsf{Let}\;\;u=x\ln(2) \implies \frac{\text{d}u}{\text{d}x}=\ln(2)`

`\textsf{Let}\;\;v=y \implies \frac{\text{d}v}{\text{d}x}=1\cdot \frac{\text{d}y}{\text{d}x}`

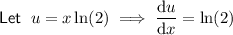
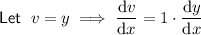
Note: Use the chain rule to differentiate terms in y only. In practice, this means differentiate with respect to y, and place dy/dx at the end.

Therefore:

`\frac{\text{d}}{\text{d}x}\left(xy\ln(2)\right)=x\ln(2) \cdot \frac{\text{d}y}{\text{d}x}+y\ln(2)`

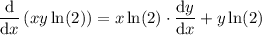
So, the final differentiated equation is:

`x\ln(2) \cdot \frac{\text{d}y}{\text{d}x}+y\ln(2)=0`

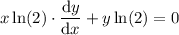
Rearrange the resulting equation to isolate dy/dx:

`x\ln(2) \cdot \frac{\text{d}y}{\text{d}x}=-y\ln(2)`

`\frac{\text{d}y}{\text{d}x}=(-y\ln(2))/(x\ln(2))`

`\frac{\text{d}y}{\text{d}x}=-(y)/(x)`

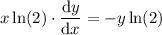
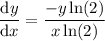
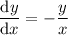
Therefore, the derivative of the given equation is:

`\large\boxed{\boxed{\frac{\text{d}y}{\text{d}x}=-(y)/(x)}}`