Question

Suppose that when your friend was born, your friend's parents deposited $9000 in an account paying %6.6 interest compounded . What will the account balance be after 13 years

Answer 1

We are given the following information

Deposited amount = P = $9000

Interest rate = r = 6.6% = 0.066

Compounding interval = n = quarterly = 4

Number of years = t = 13

We are asked to find the accumulated amount (or ending balance)

Recall that the compound interest formula is given by

`A=P(1+(r)/(n))^(n\cdot t)`

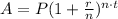
Where

A = Accumulated amount (or ending balance)

P = Deposit amount

r = Interest rate in decimal

n = Number of compounding in a year

t = Number of years

Now let us substitute the given values into the above formula

`\begin{gathered} A=P(1+(r)/(n))^(n\cdot t) \\ A=9000\cdot(1+(0.066)/(4))^(4\cdot13) \\ A=9000\cdot(1+0.0165)^(52) \\ A=9000\cdot(1.0165)^(52) \\ A=\$21077.85 \end{gathered}`

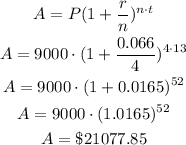
Therefore, after 13 years, the account balance will be $21077.85