Brent and Tisha both found a slope of 2, and the triangles formed by connecting the points are similar. Congruence of triangles is not guaranteed.
Certainly! Let's calculate the slope for both Brent and Tisha using the given points.
**Brent's Calculation:**
Brent used the points (-1, -3) and (0, -1). The slope (m) is given by the formula:
![\[ m = (y2 - y1)/(x2 - x1) \]](https://img.qammunity.org/2024/formulas/mathematics/college/lxpdmlkcy8rvrh1np0gxq66rh3w7b6q40f.png)
Substitute the values:
![\[ m_{\text{Brent}} = ((-1) - (-3))/((0) - (-1)) = (2)/(1) = 2 \]](https://img.qammunity.org/2024/formulas/mathematics/college/evjms0atnwkejf9fnoppwhwxkh2m6hxyhe.png)
So, Brent found a slope
 of 2.
of 2.
**Tisha's Calculation:**
Tisha used the points (3, 5) and (0, -1). Similarly, calculate the slope
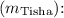
![\[ m_{\text{Tisha}} = ((-1) - 5)/((0) - 3) = (-6)/(-3) = 2 \]](https://img.qammunity.org/2024/formulas/mathematics/college/njbpsxfuurgjeqbhv467qc2djj64p7079b.png)
So, Tisha also found a slope
 of 2.
of 2.
Now, let's analyze the given options:
1. **The ratio (y2 - y1) / (x2 - x1) will be the same for Brent and Tisha:**
- True, as shown in the calculations, both have a slope of 2.
2. **Both students should find a slope of 2:**
- True, as demonstrated above.
3. **The triangles drawn between each pair of points are congruent:**
- False. Congruent triangles require equal sides and angles, and this is not guaranteed.
4. **The triangles drawn between each pair of points are similar:**
- True. Since both students found a slope of 2, the triangles formed are similar.