Question

Three vertices of a parallelogram are shown in the figure below. Give the coordinates of the fourth vertex.

Coordinates: (-3,8) (4,5) (2,-5)

Answer 1

Given:

The three vertices of a parallelogram are (-3,8), (4,5), (2,-5).

To find:

The fourth vertex of the parallelogram.

Solution:

Let the vertices of the parallelogram are A(-3,8), B(4,5), C(2,-5) and D(a,b).

We know that, diagonals of a parallelogram bisect each other. It means midpoints of both diagonals are same.

Midpoint formula:

`Midpoint=\left((x_1+x_2)/(2),(y_1+y_2)/(2)\right)`

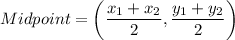
Two diagonals of ABCD are AC and BD.

Midpoint of AC = Midpoint of BD

`\left((-3+2)/(2),(8-5)/(2)\right)=\left((4+a)/(2),(5+b)/(2)\right)`

`\left((-1)/(2),(3)/(2)\right)=\left((4+a)/(2),(5+b)/(2)\right)`

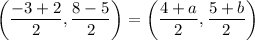
On comparing both sides, we get

`(4+a)/(2)=(-1)/(2)`

`4+a=-1`

`a=-1-4`

`a=-5`

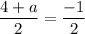
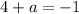
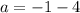
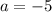
And,

`(5+b)/(2)=(3)/(2)`

`5+b=3`

`b=3-5`

`b=-2`

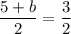
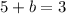
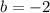
Therefore, the coordinates of fourth vertex are (-5,-2).