Question

What is the jumper's speed at this instant, when the tension is greatest in the cords? Design a "bungee jump" apparatus for adults. A bungee jumper falls from a high platform with two elastic cords tied to the ankles. The jumper falls freely for a while, with the cords slack. Then the jumper falls an additional distance with the cords increasingly tense. Assume that you have cords that are 10 m long, and that the cords stretch in the jump an additional 23 m for a jumper whose mass is 120 kg, the heaviest adult you will allow to use your bungee jump (heavier customers would hit the ground).

Answer 1

The jumper's speed at the instant when the tension is greatest in the cords is approximately
`\(14 \, \text{m/s}\)`.

To determine the jumper's speed at the instant when the tension is greatest in the cords, we can use the principle of conservation of energy. At the point of greatest tension, all the potential energy the jumper initially had will be converted into elastic potential energy stored in the stretched bungee cords.

The potential energy lost by the jumper as they fall is given by
`\(mgh\)`, where:

-
`\(m\)` is the mass of the jumper (120 kg),

-
`\(g\)`is the acceleration due to gravity (approximately 9.8 m/s²),

-
`\(h\)` is the distance fallen.

The elastic potential energy stored in the stretched cords is given by
`\(0.5kx^2\),` where:

-
`\(k\)` is the spring constant of the bungee cords,

-
`\(x\)` is the amount the cords are stretched.

Setting these two energies equal to each other and solving for the velocity
`(\(v\))` at the point of greatest tension:

`\[ mgh = 0.5kx^2 \]`

The spring constant
`(\(k\))` can be calculated using Hooke's Law:
`\(k = (F)/(x)\)`, where:

-
`\(F\)` is the force exerted by the bungee cords (equal to the jumper's weight at maximum stretch,
`(\(mg\))`,

-
`\(x\)`is the additional distance the cords stretch (23 m).

`\[ k = (mg)/(x) \]`

Now, substitute
`\(k\)` back into the energy equation and solve for
`\(v\)`:

`\[ mgh = 0.5 \left( (mg)/(x) \right) x^2 \]`

`\[ v = √(2gh) \]`

Given values:

`\(m = 120 \, \text{kg}\)`,

`\(g = 9.8 \, \text{m/s}^2\)`,

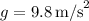`\(h = 10 \, \text{m}\)` (initial free fall distance).

`\[ v = √(2 * 9.8 * 10) \]`

`\[ v \approx 14 \, \text{m/s} \]`

Therefore, the jumper's speed at the instant when the tension is greatest in the cords is approximately
`\(14 \, \text{m/s}\)`.