Given:
The base length is 6 ft, the base height is 5 ft, and the prism height is 7 ft.
To find:
The volume of the triangular prism.
Solution:
Base of a triangular prism is a triangle with base length 6ft and height 5 ft.
Area of a triangle is
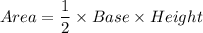
So, the area of the base is
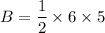
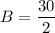
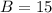
Now, the volume of a triangular prism is
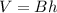
Where, B is the base area and h is the height of the prism.
Putting B=15 and h=7, we get
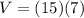
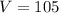
Therefore, the volume of the triangular prism is 105 cubic ft.