Answer:
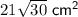
Explanation:
The formula for calculating the area of a triangle is:

In the given right triangle:
Substitute the base and height into the area formula:
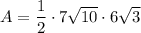
Apply the commutative property of multiplication, which states that when you multiply numbers, you can change the order of them without changing the result.

Multiply the numbers 1/2 and 6:
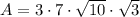
Multiply the numbers 3 and 7:
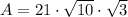

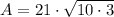
Multiply the numbers 10 and 3:
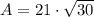
Therefore, the area of the triangle as a surd in its simplest form is:
