Question

If logx = -5, what is x?A. -0.00001B. 0.00001C. 0.00005D. -0.00005

Answer 1

Hello there. To solve this question, we have to remember some properties about logarithms.

Given the logarithmic equation:

`\log(x)=-5`

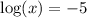
We want to determine the value of x.

For this, remember the following rule:

`\text{ For }a,\,b\in\mathbb{R}^+\text{ and }b\cancel{=}1,\text{ }\log_b(a)=c\Rightarrow a=b^c`

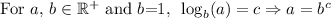
Such that, in this case, the logarithm has base 10, therefore

`x=10^(-5)`

This power of 10 can be easily found :

`x=0.00001`

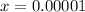
It has 5 digits after the decimal place, being the fifth digit a 1.

This is the answer contained in the option B.