Question

What is the length of side x?

Answer 1

Given:-

• A right angled triangle with two angles 60° and 30° is given to us.
• Length of two shorter sides is x and √10 .

To find:-

• The value of x .

Answer:-

Here since the given triangle is a right angled triangle, we may use the trigonometric ratios . We can see that perpendicular and base are involved in this question whose measures are "x" and "√10" respectively with respect to 60° angle. So here we may use the ratio of tangent as , in any right angled triangle,

`\implies\tan\theta =(p)/(b) \\`

and here p = x , and b = √10 . So on substituting the respective values, we have;

`\implies \tan\theta = (x)/(√(10)) \\`

Also the angle here is 60° , so that;

`\implies\tan60^o =(x)/(\sqrt10) \\`

The value tan60° is √3 , so we have;

`\implies \sqrt3 =(x)/(\sqrt10)\\`

`\implies x =√(10)\cdot √(3)\\`

`\implies x =√(10\cdot 3) \\`

`\implies x =√(30) \\`

The value of √30 is approximately 5.48 , so that;

`\implies \underline{\underline{\red{\quad x = 5.48\quad }}} \\`

Therefore the value of x is approximately 5.48 .

Answer 2

The length of side x in simplest radical form is
`√(30)`.

Explanation:

From inspection of the given right triangle, we can see that the interior angles are 30°, 60° and 90°. Therefore, this triangle is a 30-60-90 triangle.

A 30-60-90 triangle is a special right triangle where the measures of its sides are in the ratio 1 : √3 : 2. Therefore, the formula for the ratio of the sides is b: b√3 : 2b where:

• b is the shortest side opposite the 30° angle.
• b√3 is the side opposite the 60° angle.
• 2b is the longest side (hypotenuse) opposite the right angle.

We have been given the side opposite the 30° angle, so:

`\implies b=√(10)`

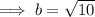
The side labelled "x" is the side opposite the 60° angle, so:

`\implies x=b√(3)`

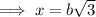
Substitute the found value of b into the equation for x:

`\implies x=√(10)√(3)`

`\textsf{Apply the radical rule:} \quad √(a)√(b)=√(ab)`

`\implies x=√(10 \cdot3)`

`\implies x=√(30)`

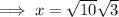
Therefore, the length of side x in simplest radical form is
`√(30)`.

`\hrulefill`

We can also calculate the length of side x using the tangent trigonometric ratio:

`\boxed{\tan \theta=\sf (O)/(A)}`

where:

• θ is the angle.
• O is the side opposite the angle.
• A is the side adjacent the angle.

From inspection of the given right triangle:

• θ = 30°
• O = √(10)
• A = x

Substitute these values into the formula:

`\implies \tan 30^(\circ)=(√(10))/(x)`

`\implies (√(3))/(3)=(√(10))/(x)`

`\implies x√(3)=3√(10)`

`\implies x=(3√(10))/(√(3))`

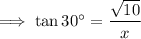
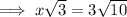
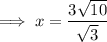
Rationalise the denominator by multiplying the numerator and denominator by √3:

`\implies x=(3√(10))/(√(3))\cdot (√(3))/(√(3))`

`\implies x=(3√(10)√(3))/(3)`

`\implies x=√(10)√(3)`

`\implies x=√(10\cdot3)`

`\implies x=√(30)`

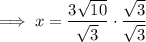
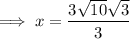
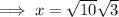
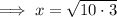
Therefore, the length of side x in simplest radical form is
`√(30)`.