Question

Given right triangle ABC with altitude BD drawn to hypotenuse AC. If AD = 33

and DC = 13, what is the length of BD in simplest radical form?

Answer 1

The length of BD in its simplest radical form is
`\sf √(429)`.

Explanation:

Geometric Mean Theorem - Altitude Rule

The altitude drawn from the vertex of the right angle perpendicular to the hypotenuse separates the hypotenuse into two segments. The ratio of the altitude to one segment is equal to the ratio of the other segment to the altitude.

`\boxed{\sf (altitude)/(segment\:1)=(segment\:2)/(altitude)}`

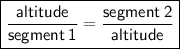
Given values (see attached diagram):

• Altitude = BD = x
• Segment 1 = AD = 33
• Segment 2 = DC = 13

To find the length of the altitude BD, substitute the values into the formula and solve for x:

`\implies \sf (BD)/(AD)=(DC)/(BD)`

`\implies \sf (x)/(33)=(13)/(x)`

`\implies \sf x^2=429`

`\implies \sf x=√(429)`

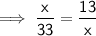
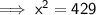
As the square root of 429 cannot be simplified any further, the length of BD in its simplest radical form is
`\sf √(429)`.