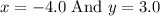Answer:
(-4,3)
Explanation:
Converting to slope-intercept form each:
x + 4y = 8
Shift 'x' term to the right side:
4y = -1x + 8
Now, divide right with the coefficient of y:
y = (-1x + 8)/4
y = (-1/4)x (+ 8/4)
Slope Intercept Form
y = -0.25x + 2
3x + 4y = 0
Shift 'x' term to the right side:
4y = -3x + 0
Now, divide right with the coefficient of y:
y = (-3x + 0)/4
y = (-3/4)x (+ 0/4)
Slope Intercept Form
y = -0.75x + 0
Now to solve for the system of:
- y = -0.25x + 2
- y = -0.75x + 0
Solve using substitution method.
The substitution method is a way to simplify using the expression of a singular variable in terms of another, thus removing the variable from the equation.
1) Solve y = -0.25+2 for y.
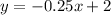
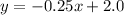 (Simplify)
(Simplify)
 ( Add (-y) on both sides)
( Add (-y) on both sides)
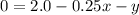
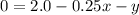 (Simplify)
(Simplify)
 ( Add (y) on both Sides )
( Add (y) on both Sides )
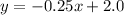
 (Divide through by 1)
(Divide through by 1)
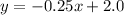
2) Substitute
 for y in y = -0.75x+0, then solve for x.
for y in y = -0.75x+0, then solve for x.
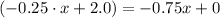
 (Simplify)
(Simplify)
 (Add (-2.0) on both sides)
(Add (-2.0) on both sides)
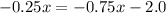
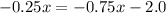 (Simplify)
(Simplify)
 (Add (0.75x) on both sides)
(Add (0.75x) on both sides)
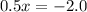
 ( Divide through by 0.5 )
( Divide through by 0.5 )

3) Substitute -4.0 for x in y = -0.25x + 2.0.
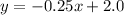
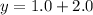
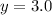
Answer