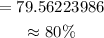Step 1
Given;
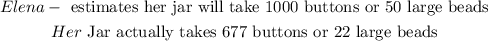
Required; To find if Elena's estimates have percentage error, to which percent, and how much less
Step 2
State the formula for percentage error
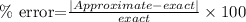
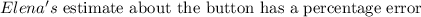
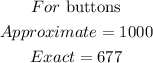

![\begin{gathered} For\text{ large beads} \\ \operatorname{\%}\text{error=}\frac{\text{\lvert50-22\rvert}}{22}*100 \end{gathered}]()
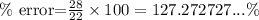
Percent errors tells you how big your errors are when you measure something in an experiment. Smaller values mean that you are close to the accepted or real value. For example, a 1% error means that you got very close to the accepted value, while 45% means that you were quite a long way off from the true value.
The percentage error for buttons with about 47.71% is less than that of the large beads which is about 127.273%.
How much less of the percentage error to the nearest percent will be;