Putting the important informations, we want to use all items and divide them into equal groups in a way that we get the most prizes.
We we want a factor that is common to the three quantities, 54, 81 and 18, and is the greatest of them.
This is a question of Greatest Common Factor or Greatest Common Divisor (different names, same thing).
To calculate it, we have to find all the common factors of theses numbers.
One way to do that is to look for numbers that can divide all of them.
The numbers are 54, 81 and 18. As we can see the three numbers are divisable by 3:
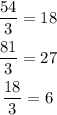
So, we now that 3 is a common factor. Let's note it to use later on.
Now have got 18, 27 and 6. We can see that, again, all of them are divisable by 3:
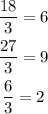
And let's note the "3" again to use later on.
The numbers now are 6, 9 and 2. 2 is only divisable by 2, but 9 isn't, so we don't have any more common factors.
In the end, we have the factor 3 and 3, which makes 3*3 = 9. Thus, 9 is the Greates Common Factor of 54, 81 and 18 and it divides them into 6, 9 and 2.
These are the answers we are looking for, because now we know that the most groups we can divide the items into is 9 and each group will have 6, 9 and 2 of those items.
So the phrase of the answer is:
"You can make at most 9 door prizes. Each door prize would have 6 packages of peanuts, 9 fruit bars, and 2 CDs."