Question

There are 23 students in a class, and 6 of them will be chosen to go on a field trip. How many ways can these students be chosen?

Answer 1

To find how many ways a group of 23 students can be chosen from a group of 6, we use combinations, where the order doesn't matter.

Combinations are found with the next formula:

`\text{nCr}=(n!)/(r!(n-r)!)`

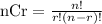
Where n is the total of persons and r is the sample asked.

Therefore:

n=23 and r=6

Replacing the values:

`23C6=(23!)/(6!(23-6)!)`

Then:

`23C6=100947`

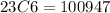
Hence, there are 100947 ways that 23 students can be chosen from a group of 6.