Question

A ball is launched at an angle of 30° above the horizontal with an initial velocity of 8 m/s. At what time is its vertical velocity 0ms?

Answer 1

0.408 seconds

Step-by-step explanation:

Assuming negligible air resistance, we can use the following kinematic equations to solve this problem:

Vertical motion:

`v_y = v_(y0) + a_y * t`

`y = y_0 + v_(y0) * t + 0.5 * a_y * t^2`

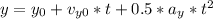
where

`v_y` = vertical velocity at time t

`v_(y0)` = initial vertical velocity = 8 m/s * sin(30°) = 4 m/s

`a_y`= acceleration due to gravity = -9.8 m/s^2 (negative because it's directed downward)

y = vertical position at time t

`y_0` = initial vertical position = 0 (assuming the ball is launched from the ground)

We want to find the time at which the vertical velocity is 0 m/s, so we can set
`v_y` = 0 and solve for t:

0 = 4 - 9.8 * t

t = 4 / 9.8 = 0.408 seconds

Therefore, the vertical velocity of the ball will be 0 m/s at 0.408 seconds after it is launched.