Question

Given f(x) =x^2+2x-15 and g(x) =3x, find the domain and range of [f o g] (x)

Answer 1

Domain:

`-\infty < x < \infty` or in interval notation
`(-\infty, \infty)`

Range:

`f(x) \ge -16` or in interval notation
`[-16, \infty)`

Explanation:

We are given

`f(x) =\:x^2+2x\:-15, \:g\left(x\right)\:=\:3x`

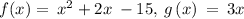
To find
`f \circ g)(x)`:

Substitute 3x wherever there is an x term in f(x)

`(f\circ g)(x) = (3x)^2 +2(3x) - 15\\\\= 9x^2 + 6x - 15`

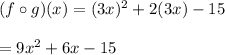
There is no restriction on the value of x so x is the set of all real numbers which means -∞ < x < ∞

`\mathrm{Domain\:of\:}\:9x^2+6x-15\:: (-\infty, \infty)` in interval notation

To find the range, note that the equation
`9x^2 + 6x - 15` is the equation of a parabola

The general parabola equation in polynomial form is

`y = ax^2+bx+c`

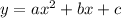
Comparing
`9x^2 + 6x - 15 \;with\;ax^2 + bx + c`

we see
a = 9
b = 6
c = -15

The vertex of this parabola is either minimum or maximum depending on the value of a

If a > 0 then it is a upward facing parabola and the vertex is a minimum
If a < 0 then it is a downward facing parabola and the vertex is a maximum

Since a = 9 > 0 it is a upward facing parabola and the vertex is a minimum
The x-coordinate of the vertex is given by

`x_v = -(b)/(2a) = -(6)/(2\cdot 9) = -(6)/(18) = -(1)/(3)`

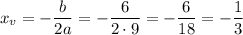
The y-coordinate of the vertex can be found by plugging in this value of x into the equation

`y_v=9\left(-(1)/(3)\right)^2+6\left(-(1)/(3)\right)-15\\\\= 9\left((1)/(9)\right) -2 - 15\\\\= 1 -2 - 15\\\\= -16`

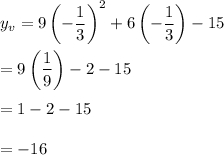
This would be the minimum value of f(x). There is no limit to the upper value of f(x) so the range is given by [-16, ∞)
Range of
`9x^2 + 6x - 15: -16 \le x < \infty` or
`f(x) \ge -16`

In interval notation this would be
[-16, ∞ )