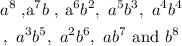Question

In the expansion of (3a + 4b)^8, which of the following are possible variable terms?

Answer 1

Step-by-step explanation:

Remember the Binomial Theorem:

`(a+b)^n\text{ =}\sum_{i\mathop{=}0}^n\begin{bmatrix}{n} & \\ {i} & {}\end{bmatrix}a^{(n\text{ - i})}b^i`

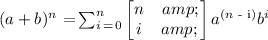
Now, consider the following polynomial:

`\left(3a+4b\right)^8`

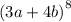
Applying the Binomial Theorem, where:

a = 3a

b= 4b

we get:

`(3a+4b)^8\text{ =}\sum_{i\mathop{=}0}^8\begin{bmatrix}{8} & \\ {i} & {}\end{bmatrix}3a^{(8\text{ - i})}4b^i`

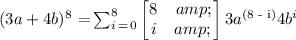
thus, expanding the sum, we get:

`\begin{gathered} \frac{8!}{0!(8\text{ -0})!}(3a)^8(4b)^0+\frac{8!}{1!(8\text{ -1})!}(3a)^7(4b)^1+\frac{8!}{2!(8\text{-2})!}(3a)^6(4b)^2 \\ +\frac{8!}{3!(8\text{ - 3})!}(3a)^5(4b)^3\text{ + ........+}\frac{8!}{8!(8\text{ -8})!}(3a)^0(4b)^8 \end{gathered}`

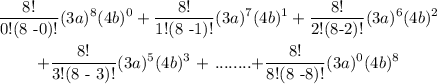
Now, simplifying we get:

`\begin{gathered} 6561a^8\text{ + 6998a}^7b\text{ + 326592a}^6b^2+870912a^5b^3+1451520a^4b^4 \\ +1548288a^3b^5+1032192a^2b^6+393216ab^7+65536b^8 \end{gathered}`

then, we can conclude that the correct answer is:

Answer:

The variable terms are:

`\begin{gathered} a^8\text{ ,a}^7b\text{ , a}^6b^2,\text{ }a^5b^3,\text{ }a^4b^4 \\ ,\text{ }a^3b^5,\text{ }a^2b^6,\text{ }ab^7\text{ and }b^8 \end{gathered}`