ANSWER
k = 1 or 21
Explanation:
According to the question, we were given the below trigonometric function
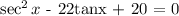
Recall that, we have trigonometric identity which is written below
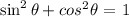
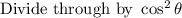
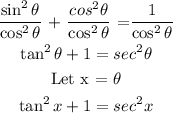
The next thing is to rewrite the equation
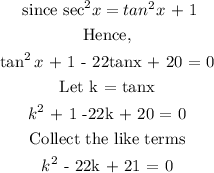
The next thing is to find the value of P by factorizing the above equation.
Recall that, the standard form of the quadratic function is given as

Let
a = 1
b = -22
c = 21
The next thing is to find the value of ac
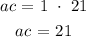
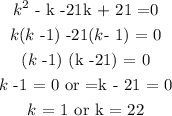
Hence, the value of k is 1 or 21