Answer:
Option A.
Explanation:
A 30° - 60° - 90° triangle is a Right Triangle that has special side measures.
Let's summarize the sides in a ratio.
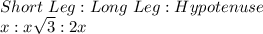
The short leg is just x.
The long leg is multiplied by
 .
.
The hypotenuse is double of the short leg.
For example, if the short leg is 2;
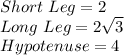
Let's look at the 4 options provided. We should check if the values of the sides match with a 30° - 60° - 90° triangle.
Option A has a short leg with the value of 5.
The long leg is correct, it's multiplied by
 .
.
The hypotenuse is correct, it's double of the short leg.
Option A is correct!
Option B has a short leg with the value of 5.
The long leg is correct, it's multiplied by
 .
.
The hypotenuse is incorrect, it's triple of the short leg.
Option B is incorrect.
Option C has a short leg with the value of 5.
The long leg is incorrect, it's multiplied by
 .
.
The hypotenuse is correct, it's double of the short leg.
Option C is incorrect.
Option D has a short leg with the value of 10.
The long leg is incorrect, it's multiplied by
 .
.
The hypotenuse is incorrect, it's
 of the short leg.
of the short leg.
Option D is incorrect.
Our only 30° - 60° - 90° triangle is Option A.