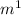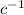Question

An enzyme that follows Michaelis-Menten (steady-state) kinetics has a KM of 10 μM and a maximum velocity of 2 μM/sec. For this enzyme, what is the initial velocity when substrate concentration is equal to 6 μM? Give your answer in units of μM/sec as a number only to 2 decimal places. If the total enzyme concentration is 8 μM, what is the specificity constant for this enzyme? Give your answer in units of μM-1sec-1 as a number only to 3 decimal places.

Answer 1

The initial velocity at a substrate concentration of 6 µM is 0.75 µM/sec. The specificity constant for this enzyme, given the total enzyme concentration of 8 µM, is 0.025 µ
`m^(-1)`
`c^(-1)`

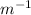
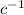
Step-by-step explanation:

The initial velocity (v) of an enzyme reaction at a given substrate concentration ([S]) when following Michaelis-Menten kinetics can be calculated using the Michaelis-Menten equation:

v = (Vmax * [S]) / (Km + [S])

Given a Km of 10 µM, a Vmax of 2 µM/sec, and a substrate concentration [S] of 6 µM, the initial velocity v is:

v = (2*6) / (10+6) = 12 / 16 = 0.75 µM/sec

The specificity constant (kcat/Km), which measures enzyme efficiency, can be calculated when the total enzyme concentration ([E]) is known and is used to find the turnover number (kcat, the number of substrate molecules converted to product per enzyme molecule per second).

kcat = Vmax / [E]

Here, [E] is 8 µM, so:

kcat = 2 / 8 = 0.25
`s^(-1)`

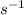
Therefore, the specificity constant is:

kcat/Km = 0.25 / 10 = 0.025 µ
`m^(1)`
`c^(-1)`