Question

Find the slope of the tangent line to the curve defined by 4x2+5xy+y4=370

at the point (−9,−1)

Answer 1

The slope of the tangent line to the curve at the given point is -11/7.

Explanation:

Differentiation is an algebraic process that finds the gradient (slope) of a curve. At a point, the gradient of a curve is the same as the gradient of the tangent line to the curve at that point.

Given function:

`4x^2+5xy+y^4=370`

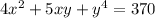
To differentiate an equation that contains a mixture of x and y terms, use implicit differentiation.

Begin by placing d/dx in front of each term of the equation:

`\frac{\text{d}}{\text{d}x}4x^2+\frac{\text{d}}{\text{d}x}5xy+\frac{\text{d}}{\text{d}x}y^4=\frac{\text{d}}{\text{d}x}370`

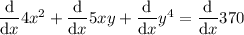
Differentiate the terms in x only (and constant terms):

`\implies 8x+\frac{\text{d}}{\text{d}x}5xy+\frac{\text{d}}{\text{d}x}y^4=0`

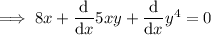
Use the chain rule to differentiate terms in y only. In practice, this means differentiate with respect to y, and place dy/dx at the end:

`\implies 8x+\frac{\text{d}}{\text{d}x}5xy+4y^3\frac{\text{d}y}{\text{d}x}=0`

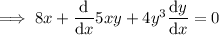
Use the product rule to differentiate terms in both x and y.

`\boxed{\frac{\text{d}}{\text{d}x}u(x)v(y)=u(x)\frac{\text{d}}{\text{d}x}v(y)+v(y)\frac{\text{d}}{\text{d}x}u(x)}`

`\implies 8x+\left(5x\frac{\text{d}}{\text{d}x}y+y\frac{\text{d}}{\text{d}x}5x\right)+4y^3\frac{\text{d}y}{\text{d}x}=0`

`\implies 8x+5x\frac{\text{d}y}{\text{d}x}+5y+4y^3\frac{\text{d}y}{\text{d}x}=0`

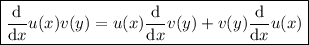
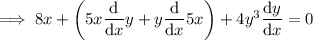
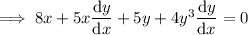
Rearrange the resulting equation in x, y and dy/dx to make dy/dx the subject:

`\implies 5x\frac{\text{d}y}{\text{d}x}+4y^3\frac{\text{d}y}{\text{d}x}=-8x-5y`

`\implies \frac{\text{d}y}{\text{d}x}(5x+4y^3)=-8x-5y`

`\implies \frac{\text{d}y}{\text{d}x}=(-8x-5y)/(5x+4y^3)`

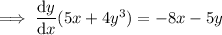
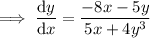
To find the slope of the tangent line at the point (-9, -1), substitute x = -9 and y = -1 into the differentiated equation:

`\implies \frac{\text{d}y}{\text{d}x}=(-8(-9)-5(-1))/(5(-9)+4(-1)^3)`

`\implies \frac{\text{d}y}{\text{d}x}=(72+5)/(-45-4)`

`\implies \frac{\text{d}y}{\text{d}x}=-(77)/(49)`

`\implies \frac{\text{d}y}{\text{d}x}=-(11)/(7)`

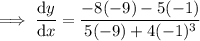
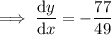
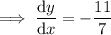
Therefore, slope of the tangent line to the curve at the given point is -11/7.