Question

A tepee in the shape of a right cone has a slant height of 18.5 feet and a diameter of 20 feet. Approximately how much canvas would be needed to cover the tepee?

Answer 1

581Answer:

Explanation:

Answer 2

To find:

The area of canvas needed to cover the tepee.

Solution:

Given that the tepee is in the shape of a right cone, with slant height 18.5 feet and diameter of 20 feet then the radius is 10 feet.

The area of canvas is equal to the curved surface area of the tepee. It is known that the curve surface area of the cone is given by:

`CSA=\pi rl`

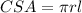
Where, r is the radius of the cone and l is the slant height of the cone. So,

`\begin{gathered} CSA=3.14*10*18.5 \\ =580.9ft^2 \end{gathered}`

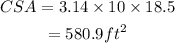
Thus, the approximate canvas that would be needed to cover the tepee is 580.9 ft^2.