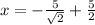Question

Rewrite the equation by completing the square - 4x^2 +20x +25 =0

Answer 1

`\left(x-(5)/(2)\right)^2=(25)/(2)`

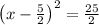
Explanation:

I am not completely sure if you just wanted me to write it in square form, so I will show the process of writing it in square form and how to find the solution.

`-4x^2+20x=-25` start by moving 25 to the right side

`(-4x^2+20x)/(-4)=(-25)/(-4)` divide both sides by -4

=
`x^2-5x=(25)/(4)`

Now we rewrite the equation in the form
`\:x^2+2ax+a^2`

=
`x^2-5x+\left(-(5)/(2)\right)^2=(25)/(2)` apply perfect square formula:
`\left(a-b\right)^2=a^2-2ab+b^2`

=
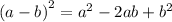
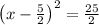`\left(x-(5)/(2)\right)^2=(25)/(2)` -- square form

Solutions to the quadratic equation:

Possible solutions:
`x=(5)/(√(2))+(5)/(2)` and
`\:x=-(5)/(√(2))+(5)/(2)`