Question

Does anyone know the answer?!?​

Answer 1

Explanation:

Lets find the slope of the line first so we can write the equation.

Counting the slope, we can see the slope of the line is
`(3)/(1)` or 3, so we have to write the equation of the line in slope intercept form
`y=mx+b` where m is the slope and b is the y intercept.

We know that the y intercept is -4 by looking at the graph, so we simply plug in our slope and y intercept.

`y=3x-4`

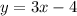
To tell if equations are parallel or perpendicular:

Parallel: The slope is the same

Perpendicular: The slope is the opposite reciprocal

Lets look at the equations and see if there parallel:

1.
`y=-3x+10` is neither.

2. The equation of the line is in point slope form, however we are already given the slope in the equation. The slope is
`-(1)/(3)`, which is the opposite reciprocal of 3, therefore it is perpendicular.

3.
`(1)/(3)` is not the opposite reciprocal of 3, it is neither.

4. The equation of the line is in standard form, which means we must solve for y to get it in slope intercept form

`-3x+y=1`
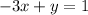
Subtract -3x on both sides

`y=1-(-3x)`

Simplify

`y=3x+1`

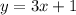
The equation has the same slope, so it is parallel.