Question

Find the measure of CD.
mCD= [?] degrees

Answer 1

Given:

A circle with radius 7 units and chord CD = 7 units.

To find:

The measure of arc CD.

Solution:

Let O be the center of the circle.

In triangle OCD,

`OC=7` (Given)

`OC=OD=7` (Radii of same circle)

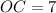`CD=7` (Given)

Since
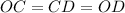`OC=CD=OD` all sides are equal, therefore, the triangle OCD is an equilateral triangle.

Measure of each angle of an equilateral triangle is 60 degrees. So,

`m\angle COD=60^\circ`

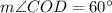
The measure of central angle is equal to the measure of corresponding arc.

`m(arcCD)=m\angle COD`

`m(arcCD)=60^\circ`

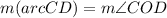
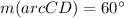
Therefore, the measure of arc CD is 60 degrees.