Question

A 98 N person is standing on a board, 1 m from the end. The board is balanced on a point that is 2m from the same end. The board is 49 N. How long is the board overall?

Answer 1

8 m

Explanation:

To calculate the length is the board overall we must apply the center of mass formula, which is as follows:

`x_(cm)=\frac{m_{\text{board}}\cdot x_{\text{noard}}+m_p\cdot x_p}{m_{\text{board}}+m_p}`

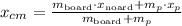
The mass of the person and that of the board, we calculate it as follows:

`\begin{gathered} F_{\text{p}}=m_{\text{p}}\cdot g_{} \\ m_{\text{p}}=\frac{F_{\text{p}}}{g}=(98)/(9.8)=10kg \\ F_{\text{board}}=m_{\text{board}}\cdot g_{} \\ m_{\text{board}}=\frac{F_{\text{board}}}{g}=(49)/(9.8)=5kg \end{gathered}`

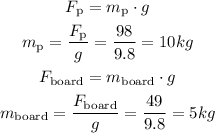
Replacing:

`\begin{gathered} -2=\frac{5\cdot x_{\text{board}}+10\cdot(-1)}{5+10} \\ (-2)(15)+10=5\cdot x_{\text{board}} \\ x_{\text{board}}=(-20)/(5) \\ x_{\text{board}}=-4\text{ m} \end{gathered}`

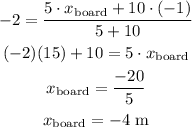
Since the CM of the board is only 4 m from the edge of the board, and the MC of the board is at its center, the board is 8 m long.