The centripetal force : F = 293.3125 N
Further explanation
Given
mass = 65 kg
v = 9.5 m/s
r = 20 m
Required
the centripetal force
Solution
Centripetal force is a force acting on objects that move in a circle in the direction toward the center of the circle
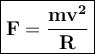
F = centripetal force, N
m = mass, Kg
v = linear velocity, m / s
r = radius, m
Input the value :
F = 65 x 9.5² / 20
F = 293.3125 N