Question

What is equation of the line graphed below?

Answer 1

using the parent function y = mx + b,
y = -3x
slope is m- so rise over run -3/1 = -3
where the line crosses the y axis is b- 0 (origin)

Answer 2

Step-by-step explanation

• Find the slope by using rise over run with two coordinate points.

`(0,0) \longrightarrow \sf{part \: \: of \: \: the \: \: graph} \\ (1, - 3)\longrightarrow \sf{given \: \:coordinate \: \: point}`

`m = (dy)/(dx) = (y_2-y_1)/(x_2-x_1)`

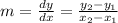
Substitute these coordinate values in.

`m = (0 - ( - 3))/(0 - 1) \\ m = (3)/( - 1) \\ m = - 3`

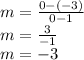
Hence, the slope is -3.

• Find the y-intercept.

From the graph, the line passes through the origin point. Therefore, the y-intercept is (0,0).

• Slope-Intercept form

`y = mx + b \\ \begin{cases} \sf{m = slope} \\ \sf{b = y - intercept} \end{cases}`

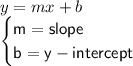
Substitute both m and b in the slope-intercept form.

`y = - 3x + 0 \\ y = - 3x`

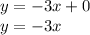
Hence the answer is y = -3x.

Answer

`\large \boxed{y = - 3x}`