The solution of a system of inequalities is the region in which the regions of the inequalities that make up the system intersect.
For the first inequality, its graph will be a vertical line with ordered pairs (-4, a), where a is any number. Also, a dashed line is graphed because x never becomes -4. Then, the region to be graphed will be all values less than x = -4. So,
For the second inequality, its graph will be a horizontal line with ordered pairs (b, -8), where b is any number. Also, a continuous line is graphed because Y if it can be -8. Then, the region to be plotted will be all values greater than y = -8. So,
For the third inequality, you can first solve for y from the inequality, like this
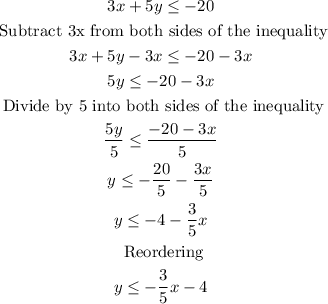
Now, you can graph a line with a slope equal to -3/5 and y-intercept equal to -4. Also, a continuous line is graphed because the symbol is less than equal. Then, the region to be graphed will be all the ordered pairs in which the Y coordinate is less than or equal to the line that you just graphed.
Finally, the graph of the solution of the system of inequalities is the region in which the regions of the inequalities that make up the system intersect.