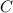Question

Why is it not possible to make a right triangle using lengths of 4 feet, 8 feet, and 10 feet? A. 4 + 8 is greater than 10.B. 10 - 8 does not equal 4.C. 4^2 + 8^2 does not equal 10^2which is the answer?A.B.C.

Answer 1

If three sides are the sides of a right triangle, then the greatest side is the hypotenuse and the lengths should satisfy the Pyhtagorean Theorem:

`a^2+b^2=c^2`

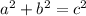
Notice that:

`\begin{gathered} 4^2+8^2=16+64=80 \\ 10^2=100 \end{gathered}`

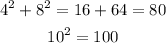
Since:

`4^2+8^2\\e10^2`

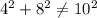
Then, 4, 8 and 10 cannot be the sides of a right triangle.

Therefore, the answer is:

`C`