Question

Find the slope of the line

Answer 1

`(3)/(2)`

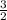
Explanation:

For this problem, we are asked to find the slope of the given line.

What is slope?

The slope is the change within a line. Think of slope as a measurement of steepness, the greater the rise (y), the more steeper the line is. However, the greater the run (x), the line will become less steeper.

Let's begin by reviewing the slope formula. The slope formula is made up as a fraction subtracting 2 points from a given line. The difference between these 2 points will result in the slope, only shown as a fraction. See below.

Formula:

`(y_(2) - y_(1) )/(x_(2) - x_(1) ) = m \ (slope)`

Points:

• (-2, -4)
• (2, 2)

Let's substitute the points into our slope formula.

`(2 + 4)/(2 + 2) =m`

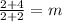
Simplify:

`(6)/(4) =m`

`(3)/(2) =m`

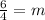
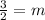
The slope of the given line is
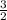`(3)/(2)`.

Answer 2

To find the slope, we follow the formula of rise (as the numerator, y) over run (as the denominator, x) from the left to right points.

To get from the left to right point on this graph, we move up 6 units and to the right 4 units. Our slope is 6/4, or 3/2 as simplified.