You have to solve the following inequality:

To solve an inequality the method is similar as when you are solving an equation.
First, using addition/subtraction rearrange the terms so that all x-related terms end up in one side of the expression and the constants remain in the other side:
First add 5 to both sides of the inequality and solve:

Next subtract 5x to both sides and solve:
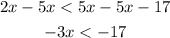
Finally you have to divide both sides by -3 to determine the possible value of x.
Be careful, when you are solving an inequality and divide it by a negative number, do not forget that this operation changes the direction of the inequality, this means that if the original inequality had the "<" symbol, after dividing by the negative number the symbol will be ">"
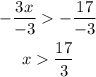
The solution of this inequality are all values greater than 17/3