Question

A ladder is leaning against a building. The ladder forms an angle of 77° with the ground. The distance from the base of the ladder to the base of the building is 8 feet.

A ladder leans against a building, forming the hypotenuse of a right triangle with the building and ground.

How high on the building does the ladder make contact? Round your answer to the nearest hundredth.

Values in the table are rounded to the ten-thousandths place.

Answer 1

34.65 feet

Explanation:

A right-angled triangle is formed where:

The height of the ladder = hypotenuse

With respect to the angle 77°:

Distance from the base of the ladder to the base of building = Adjacent

= 8 feet

h = How high on the building does the ladder make contact = Opposite

Use tanФ trigonometric function to determine h:

tanФ =
`(Opposite)/(Adjacent)`

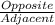
= tan77° =
`(h)/(8)`

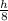
Cross-multiplication is applied:

h = (8)(tan77°)

∴h = 34.65 feet (Rounded to the nearest hundredth or 2 decimal places)