Question

A racing car consumes a mean of 98 gallons of gas per race with the standard deviation of 3 gallons. If 42 racing cars are randomly selected what is the probability that the sample mean will be greater than 98.6 gallons? Round your answer to four decimal places

Answer 1

Given:

Mean= 98

Standard Deviation= 3

Sample = 42

Observed value = 98.6

To determine the probability that the sample mean will be greater than 98.6 gallons, we first note the z-score formula:

`\begin{gathered} z=(x-\mu)/((\sigma)/(√(n))) \\ where: \\ x=observed\text{ value} \\ \mu=mean \\ \sigma=standard\text{ deviation} \\ n=sample \end{gathered}`

Next, we plug in what we know:

`\begin{gathered} z=(x-\mu)/((\sigma)/(√(n))) \\ z=(98.6-98)/((3)/(√(42))) \\ Calculate \\ z=1.2961 \end{gathered}`

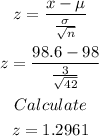
Then, we also note that:

`P(x>98.6)=P(z>1.2961)=0.0975`

Therefore, the probability that the sample mean will be greater than 98.6 gallons is: 0.0975