Question

Hi, Pls someone help!

A boat traveling with a velocity measured in meters per second is described by the vector b = <-4, 3>. In two or more complete sentences explain how to find the speed of the boat and the direction it is traveling in standard position. In your final answer, include all of your calculations.

Answer 1

Explanation:

The speed of the boat is absolute value of the velocity vector. To find the speed of the boat, take the magnitude of the velocity vector which is

`\sqrt{ {a}^(2) + {b}^(2) } = {c}^{}`

Where a and b is the vector

a is -4, b is 3

so

`√(16 + 9) = √(25) = 5`

So the speed of the boat is 5 meters per hour.

To find the direction of the vector, we need to find the angle it is traveling in.

Using trigonometry, we can do this.

`\tan( \alpha ) = - (3)/(4)`

`arc \tan( - (3)/(4) ) = \alpha`

`\alpha = - 36.87`

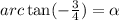
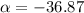
The boat is traveling at angle 36.87 degree clockwise.