Question

If a store sells each DVD for $x, the store will sell 200-8x DvDs.A) the store sold 80 DVDS yesterday What was the price of each DVD?b)the store wants to sell at least 80 DVDs. what range of prices will ensure this?c) the store want to sell at least 150 DVDS.what range of the prices will ensure this?

Answer 1

Given:

Cost of each DVD = $x

Number of DVDs sold = 200 - 8x

Let's solve for the following:

A) The store sold 80 DVDs yesterday. What was the price of each DVD?

To find the price of each DVD if 80 DVDs were sold, we have the equation:

200 - 8x = 80

Let's solve for x.

Subtract 200 from both sides:

200 - 200 - 8x = 80 - 200

-8x = -120

Divide both sides by -8:

`\begin{gathered} (-8x)/(-8)=(-120)/(-8) \\ \\ x=15 \end{gathered}`

Therefore, the price of each DVD was $15

B) The store wants to sell at least 80 DVDs. What range of prices will ensure this.

To find the range of prices, we have the inequality:

`200-8x\ge80`

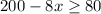
Let's solve for x.

Subtract 200 from both sides:

`\begin{gathered} 200-200-8x\ge80-200 \\ \\ -8x\ge-120 \end{gathered}`

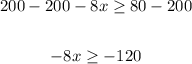
Divide both sides by -8:

`\begin{gathered} (-8x)/(-8)\ge(-120)/(-8) \\ \\ (-8x)/(-8)\leq(-120)/(-8) \\ \\ x\leq15 \end{gathered}`

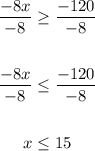
The range of prices that will ensure this must be from $0 to $15

This means the price of each DVD must not be more than $15

C) The store wants to sell at least