Question

Let X represent the full height of a certain species of tree. Assume that X has a normal distribution with a mean of 191.8 ft and a standard deviation of 4 ft. A tree of this type grows in my backyard, and it stands 182.2 feet tall. Find the probability that the height of a randomly selected tree is as tall as mine or shorter.

Answer 1

0.0082 = 0.82% probability that the height of a randomly selected tree is as tall as mine or shorter.

Explanation:

When the distribution is normal, we use the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

Assume that X has a normal distribution with a mean of 191.8 ft and a standard deviation of 4 ft.

This means that
`\mu = 191.8, \sigma = 4`

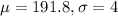
A tree of this type grows in my backyard, and it stands 182.2 feet tall. Find the probability that the height of a randomly selected tree is as tall as mine or shorter.

This is the pvalue of Z when X = 182.2. So

`Z = (X - \mu)/(\sigma)`

`Z = (182.2 - 191.8)/(4)`

`Z = -2.4`

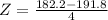
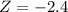
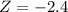`Z = -2.4` has a pvalue of 0.0082

0.0082 = 0.82% probability that the height of a randomly selected tree is as tall as mine or shorter.